Interferometric Sensing for Drag-Free Satellites
The development of drag-free technology is central to the exploitation of space as an environment for missions in Fundamental Physics. Missions such as Gravity Probe B, LISA and STEP are key space missions that exploit drag-free technology to achieve their scientific goals.
![[LISA and Gravitational Waves]](images/lisa_wave.jpg)
LISA and Gravitational Waves.
The LISA (Laser Interferometry Space Antenna) mission will open up a new window on the universe by enabling the observation of low frequency gravitational waves. It can be expected to have an impact as significant as the development of infra-red or X-ray astronomy. LISA is a joint ESA-NASA mission that involves having three spacecraft flying approximately 5 million kilometres apart in an equilateral triangle formation. Together, they act as a Michelson interferometer to measure the distortion of space caused by passing gravitational waves.
Drag-Free Control Systems
The three identical spacecraft will orbit the sun at a distance of 1 AU, trailing the Earth by 20 degrees. Proof Masses within each spacecraft are shielded from all external perturbations, so that their motion describes a perfect geodesic. These serve as optical references to the 1 Watt 1064nm laser used to measure their relative positions in space. In such missions, the key is to ensure that the proof-mass (not the surrounding spacecraft) remains in inertial space within the bandwidth required to achieve the science goal. This consideration leads naturally to a drag-free control system where the spacecraft is decoupled as much as possible from the surrounding spacecraft.
![[Drag-Free Control Loop]](images/control_loop.jpg)
Drag-Free Control Loop.
The acceleration, a, imposed on the proof-mass by the spacecraft is given as:
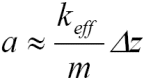
Where ![]() is the effective stiffness
or spring constant that couples the spacecraft to the proof-mass of mass, m,
and
is the effective stiffness
or spring constant that couples the spacecraft to the proof-mass of mass, m,
and ![]() is
the motion of the proof mass relative to the spacecraft. An ideal drag-free
control system will attempt to position the spacecraft so as to minimise
is
the motion of the proof mass relative to the spacecraft. An ideal drag-free
control system will attempt to position the spacecraft so as to minimise ![]() ,
resulting in a residual displacement equal in magnitude to the sensor
displacement noise,
,
resulting in a residual displacement equal in magnitude to the sensor
displacement noise, ![]() . The spectrum of proof mass
acceleration noise produced by spacecraft motion can then be shown as:
. The spectrum of proof mass
acceleration noise produced by spacecraft motion can then be shown as:
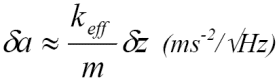
Note that additional noise sources, such as thermal noise, are not considered in this relation. Critically however, fundamental methods of realising a drag-free control system can be identified:
To minimise ![]() , it is also necessary maximise
the distance between the proof-mass and spacecraft to eliminate electrostatic
forces (e.g. patch-fields) the ultimate goal is to be limited by the
gravitational field of the spacecraft.
, it is also necessary maximise
the distance between the proof-mass and spacecraft to eliminate electrostatic
forces (e.g. patch-fields) the ultimate goal is to be limited by the
gravitational field of the spacecraft.
Capacitive sensor techniques are at present widely adopted for proof-mass position sensing. However, this solution is in trinsically unstable, since they can apply substantial forces to the proof mass and require conducting surfaces in close proximity to it. Interferometric optical sensors are a significant improvement over capacitive sensing, since they prov ide:
Furthermore, the inherent stability and accuracy offered by optical interferometers could provide drag-free operation at ultra-low frequencies. At Birmingham we have been developing a compact interferometric sensor.
Interferometric Sensor Development
We have constructed a Michelson interferometer using the homodyne technique, due to its simple design and lack of moving or active elements. With this method two or more interferometer outputs are used to accurately determine displacements with a resolution of much less than one fringe but with displacement ranges of many mm without the need for any ac modulation of optical paths or electro-optic modulators (heterodyne techniques). Each output intensity varies sinusoidally with the target mirror displacement but with phase offsets of zero, pi/2 and pi.
After constructing a bench-top demonstration interferometer we undertook to design and fabricate a compact version of th e device. The following image shows the CAD model (insert) and assembled prototype interferometer. This compact interfer ometer uses a VCSEL diode source (850nm) and approximately 100nW of optical power incident upon the photodiodes.
![[Interferometric Sensor Prototype]](images/prototype.jpg)
Interferometric Sensor Prototype.
Although very promising, a possible problem with the application of the homodyne and heterodyne interferometers is that the output at a given time is given as a sum of previous displacements and is not absolute. This has the significant drawback that:
However, it is possible to use a wavelength modulation scheme to overcome these issues.
Proof Mass Biasing and Actuation
Another consideration is the need to apply forces to bias the drag-free system for orbital manoeuvres or to position the proof mass within the spacecraft. To solve this problem and other concerns that we have raised, it will be desirable to include in the design a method of applying electrostatic forces, for example, to allow control of all degrees of freedom internally to the spacecraft and low sensitivity capacitive sensors.
A cage structure for applying forces to the drag-free proof-mass in the x and y directions, is shown below. The rods in the top and bottom horizontal planes are positioned such that they apply zero stiffness between the spacecraft and the proof mass. The rods are split into two to allow capacitive sensors to be used as a back-up to the optical sensors. The proof mass is a cube, 40mm each dimension.
![[Cage]](images/cage.jpg)
Caging Mechanism Avoids the Charging Problems Associated with Capacitive Sensors.
![[Cage]](images/displacement.png)
Capacitance vs. Displacement of the Proof Mass.
Contact
For further information contact C. C. Speake and S. M. Aston