FORCES ON MOVING CHARGES - cont'd

What is the effect of the Lorentz force on the electron in a vacuum?

     Electrons in vacuum are completely free and are therefore unrestrained in their response to the Lorentz force. The fact that the force always has to be at right angles to the velocity means that, in a uniform field, the force can only change the direction of the velocity, not increase the magnitude of the velocity. Hence it accelerates the charge but cannot impart energy to it; no work is being done on or by the charge. If the particle is a free particle then its motion is constrained to be in a circle in much the same way as a satellite orbits the Earth.

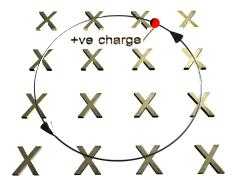
ANIMATION - A positive charge in a magnetic field

A positive charge will follow a circular path (counterclockwise) in a magnetic field

Please move mouse over image

     By equating the Lorentz force to the centripetal force it is easy to show that the radius of the circular motion (called the Larmor radius or radius of gyration) is given by

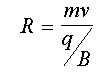
     and that the number of orbits executed per second (the Larmor frequency) is given by

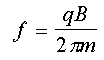
     See if you can derive these two formulae.

     Since such electrons are not bound or close to atoms they can be considered as classical particles and not subject to quantisation rules. And since these particles are being accelerated they must therefore be radiating. The frequency of the electromagnetic radiation that they emit is the same as the Larmor frequency. They lose energy as a consequence, decrease their velocity and hence the radius of gyration is reduced. However we can see that the Larmor frequency is unchanged. (How can a satellite lose energy and, if it does, does anything similar happen?)

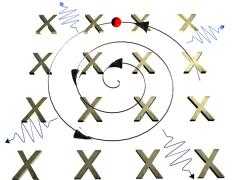
IMAGE - A free electron will follow a helical path.

A free electron unlike a bound electron will radiate its energy away. It will therefore follow a helical path as it loses energy.

What is the effect of the Lorentz force on the charged particles in space?

     Many of the atoms in space are ionised, especially if they are near a source of energy such as our Sun. When atoms are (singly) ionised the atom splits into two components. As well as the free electron that we discussed above there is also the positive ion. They have the same magnitude of charge, e, (though one is positive and the other negative), but the ion has a significantly greater mass. Both particles will be affected by the Lorentz force but in different ways. For a given velocity the electron has a smaller Larmor radius than the ion and moves in the opposite sense around the magnetic field, and it has a larger Larmor frequency than the ion. Both particles will radiate electromagnetic radiation but clearly at different frequencies.

    Please click here for animation. (Charged Particles in a Mag Field: Interactive Physics & Maths with Java).

IMAGE - The magnetic field applet.

A magnetic applet showing the motion of charged particles in a magnetic field.

Please click on image.

     Because of the direction of the force in the relation to the magnetic field, we can see that it is very difficult for a charged particle to "cross" a magnetic field line; the magnetic field acts as a barrier to transverse motion. Any velocity component along the field line does not generate a force and is therefore unaffected. Hence charged particles become attached to magnetic field lines and spiral along them.